When reading our blog posts, you might find yourself asking what the scale of the black holes, jets, or accretion disks we speak of are. If that is the case, then this post is for you!
Given that Halloween just happened, let us choose our base unit as one Jack-o’-lantern pumpkin (JOLP) that is 20 kg heavy and 0.5 meters in diameter. For scale, roughly four JOLPs stacked on top of each other would span a human that is 1.8 meters tall and 80 kg heavy.
Right, then how many JOLPs would we need to span the entire circumference of the Earth? Well, we would need about 80 million (80 million seconds is equivalent to 2.5 years)! If we placed one JOLP one in front of the other every second, all the way from the Earth to the Sun, it would take 9,500 years. Clearly pumpkins are not adequate enough to scale outer space. Using time, however, may have somewhat clarified the scale of the universe.
The current world record for the 100 meter sprint is 9.58 seconds, and it was set by Usain Bolt. This is equivalent to about 36 km every hour. If Usain Bolt were to run from one end of the Milk Way Galaxy to the other end at this pace, it would take him longer than 3,000,000,000,000 (trillion) years! Comprehending how large this number is can be a mind bender, so let us introduce the speed at which light can travel in a year (light year). Light can travel 9,461,000,000,000 km in one year. It would therefore take 105,700 years for light to travel across the Milky way from end to end, whilst Bolt would be almost 30 million times slower. We can now see the significance of the speed of light in helping us understand scales.
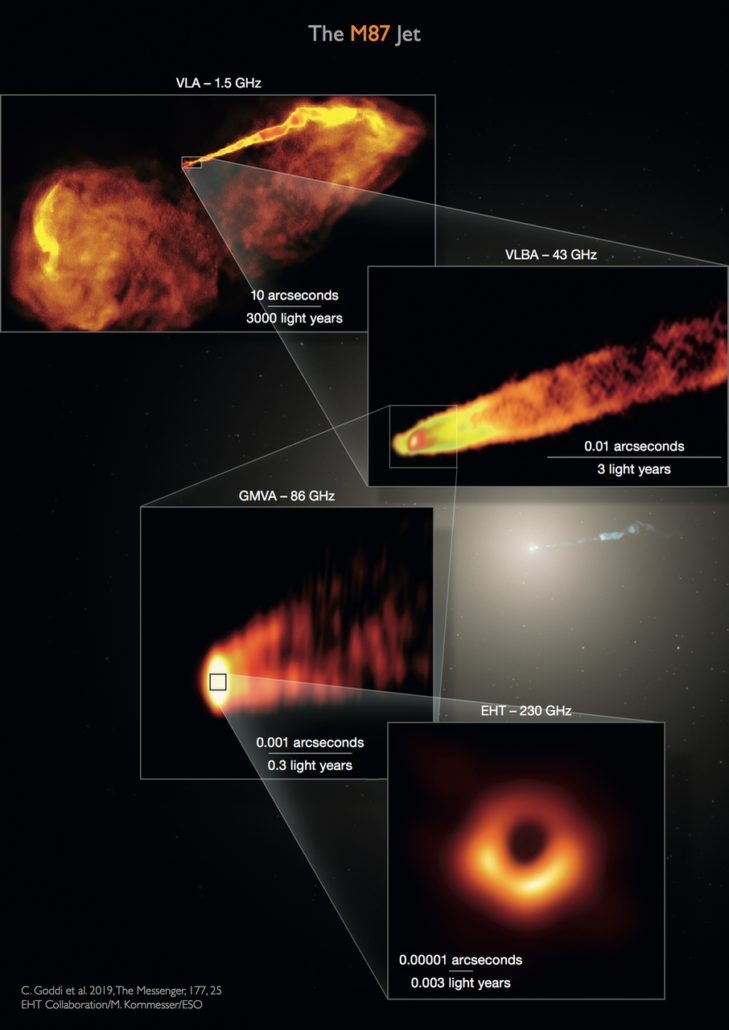
The M87 galaxy has a massive jet that is over 18,000 light years across (image credit: Goddi et al., 2019). As we zoom in from the outer region of the jet inwards, we see that the central region reveals the famous “red doughnut” image of the M87 black hole (M87*). The diameter of the black hole roughly 0.024 light years across. That is more than 450,000,000,000,000 (trillion) JOLPs across! In addition, we know that M87* is roughly 6,500,000,000 times heavier than our Sun. Calculating the mass of M87* in terms of pumpkins is clearly nonsensical.
Coming to grips with how massive astrophysical objects are can be quite a mind bender. But the physics involving these systems is even more complex and exciting. What is the black hole shadow? Where does the jet originate? Why is the ring asymmetric? These are all important questions which will be delved into in an upcoming post!